issue contents
September 2006 issue
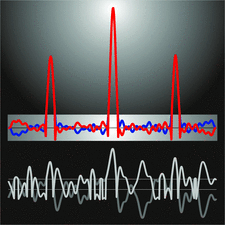
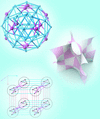
Cover illustration: Determination of a one-dimensional structure by the charge flipping algorithm. Bottom curves: grey is the initial electron density obtained with a random choice of structure-factor phases; white is the result of charge flipping, i.e. the sign of the density below a threshold is reversed. Top curves: electron density after convergence (blue) and its flipped counterpart (red). Only the three atomic regions correspond to the true structure, small oscillations do not. See Oszlányi & Süto [Acta Cryst. (2004), A60, 134-141; (2005), A61, 147-152].
research papers


 access
access

 journal menu
journal menu