issue contents
May 2024 issue

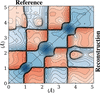
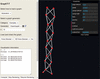
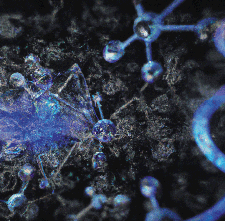
Cover illustration: In a previous paper [Acta Cryst. (2022), A78, 212–233] Day and Hawthorne used graph theoretic methods to generate topological representations of some observed and hypothetical chains of [SiO4]4− tetrahedra. In this issue Day et al. consider possible restraints on embedding these chain graphs into two- and three-dimensional Euclidean space such that they are compatible with the metrics of chains of tetrahedra observed in real crystal structures [Acta Cryst. (2024), A80, 258–281; Acta Cryst. (2024), A80, 282–292]. The two chain arrangements shown are not observed in silicates: when embedded in two dimensions, they form planar curves that force unrealistic T⋯T distances between vertices ([SiO4]4− tetrahedra). When embedded in three dimensions, they form helical arrangements which allow realistic T⋯T distances.
foundations
research papers
 access
access access
access access
access access
access access
access
 journal menu
journal menu